Complex Number Kookiness 6

(1 + i)(x + xi) = 2xi

Examples:

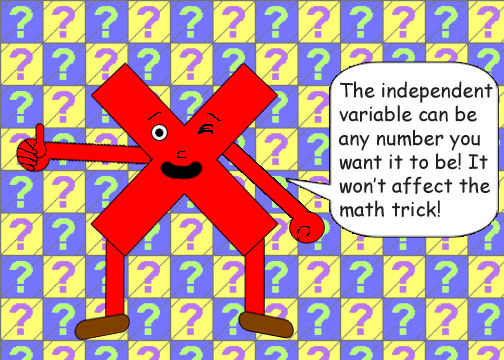
(1 + i)(2 + 2i) = 4i

(1 + i)(7 + 7i) = 14i

(1 + i)(0 + 0i) = 0i

[Remember that any number multiplied by zero(0) is still zero!]

(1 + i)(101 + 101i) = 202i

(1 + i)(√(2) + i√(2)) = 2i√(2)

(1 + i)(π + πi) = 2πi

This math trick works with all numbers; however, if you pick an imaginary or complex number for x, then... Well, look at the examples below to find out:

(1 + i)(i + i * i) = (1 + i)(i + i²) = (1 + i)(-1 + i) = -2

Let A = (1 + i); so, (1 + i)(A + Ai) = (1 + i) * 2i = -2 + 2i

Let B = (1 - i); so, (1 + i)(B + Bi) = (1 + i) * 2 = 2 + 2i

Since i² = -1, it affected the 2nd complex number in these 3 final examples. Also, the real part of a complex number is usually printed to the left of the plus or minus sign in-between. (A minus sign means that the imaginary part is negative; if you see a minus sign to the left of the real part, then the real part is negative.)

However, the final examples are still correct according to the formula at the top of this Web page!

Back to Index Page Back to Math Trick Menu