Delightful Division 3: Reciprocals of Half-Integers
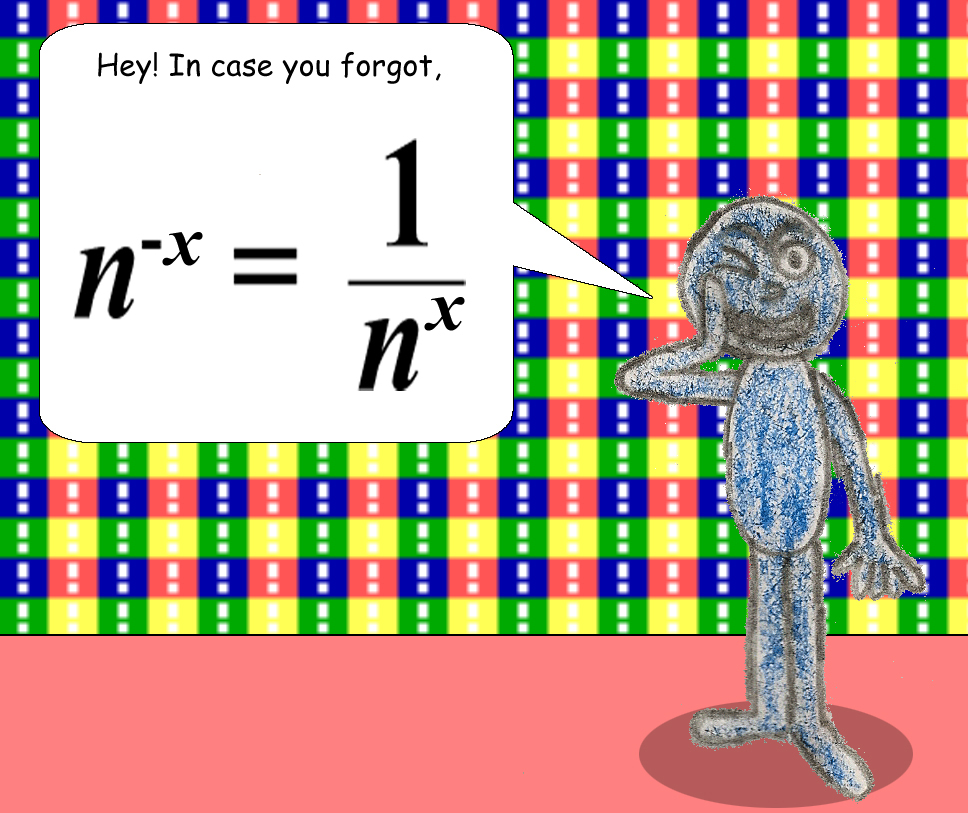
y = 1/x or x-1
This function gives you a number's reciprocal. If x is equal to a half-integer...
| x | y |
| ½ | 2/1(or simply 2) |
| 1½ | 2/3 |
| 2½ | 2/5 |
| 3½ | 2/7 |
| 4½ | 2/9 |
| 5½ | 2/11 |
| 6½ | 2/13 |
| 7½ | 2/15 |
| 8½ | 2/17 |
| 9½ | 2/19 |
| 10½ | 2/21 |
| 11½ | 2/23 |
| W + ½ | 2/(2W + 1) |
{You understand how I used the variable W in the bottom row, do you?}
All the fractions in the y column have a common numerator: 2, but have you noticed that the denominators are consecutive odd numbers? Interesting, isn't it?
Note: x/1 = x since 1 is the number of multiplicative identity. Whenever the number 1 is a denominator of a fraction, the fraction technically turns into an integer! (Algebra, calculus & wacky irrational numbers as numerators are not considered)

By the way, this same math trick happens on the negative side of the number line, if you get what I mean! It'll also work with imaginary numbers, but only real numbers are allowed in function tables.
Back to Index Page Back to Math Trick Menu
© Derek Cumberbatch


