Impossible Equations

There are functions that never intersect because they're parallel to each other. (Especially if they're linear functions, but some non-linear functions never intersect either.)

Below are some examples of non-intersecting function pairs:

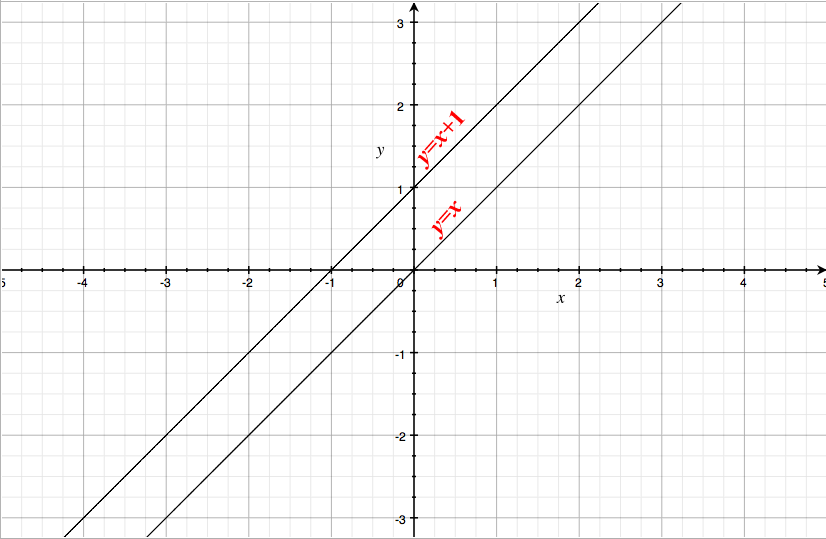
x is never equal to x + 1 because these 2 linear functions are parallel to each other.

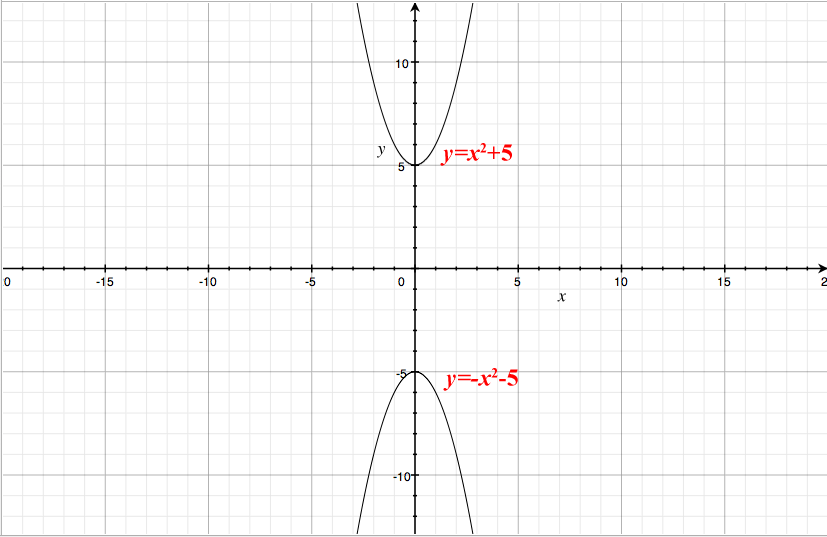
x² + 5 is never equal to -x² - 5 because these 2 quadratic functions don't intersect.

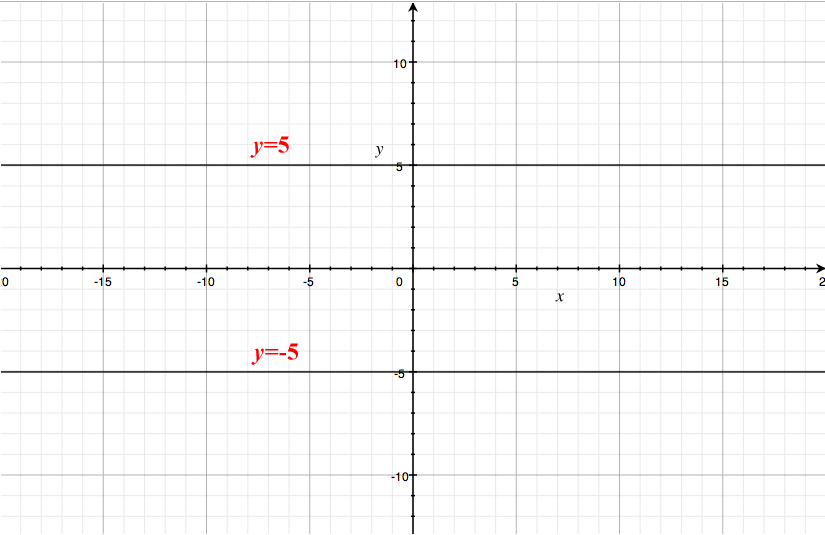
These 2 functions are constant functions. Obviously, 5 ≠ -5 because they're 2 different numbers. (But they are additive inverses.)

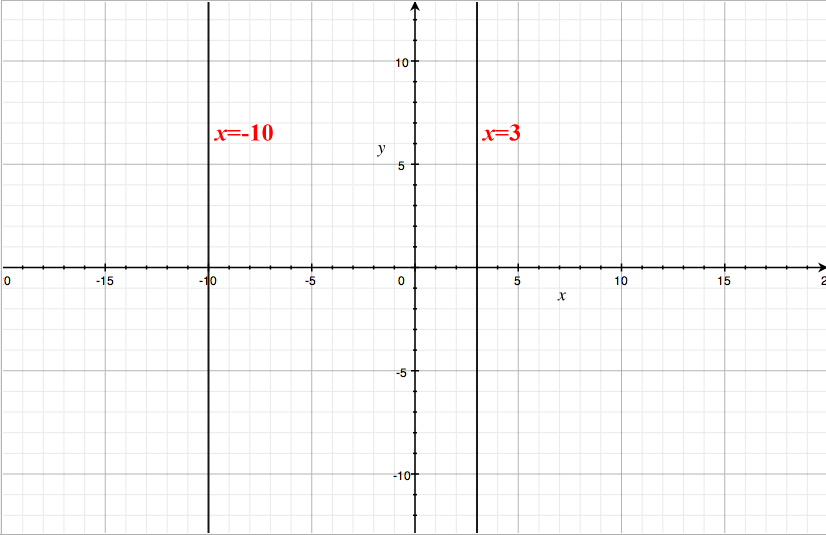
Here's a pair of vertical x-lines. This is more proof that 2 different numbers are never equal.

This is the last example I'll use on this page:

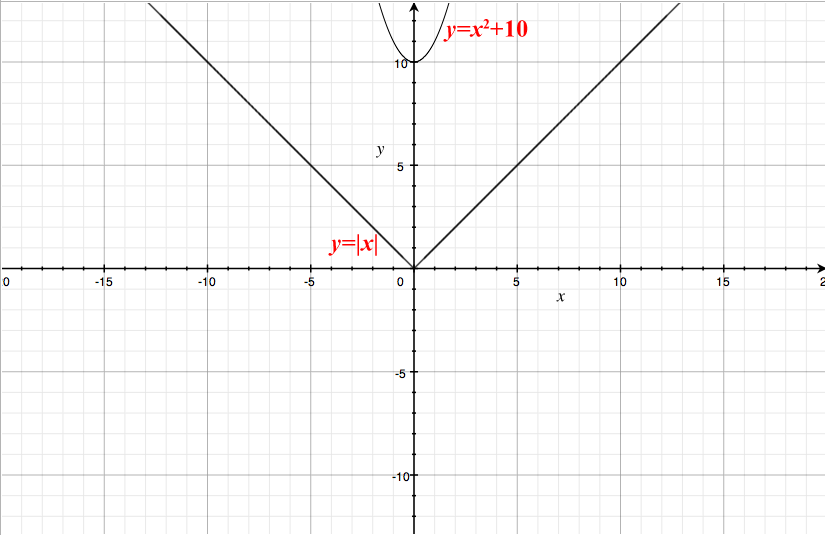
x² + 10 is never equal to |x| since the 2 functions never intersect.

2 functions don't have to be the same type of function to never intersect!

Back to Index Page Back to Math Trick Menu

© Derek Cumberbatch