Unit Fraction Uniqueness 2

When you add unit fractions with consecutive denominators, something quite interesting happens! Can you figure out the pattern to the table below? The last row will show you the formula!

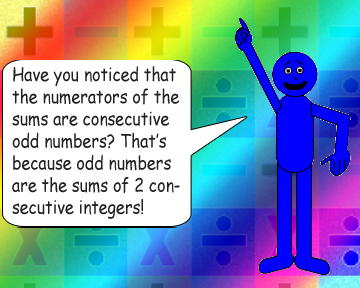
Fraction #1Fraction #2Sum
1/21/35/6
1/31/47/12
1/41/59/20
1/51/611/30
1/61/713/42
1/71/815/56
1/81/917/72
1/91/1019/90
1/x1/(x+1) (2x+1)/(x^2+x)

You get the sum of the denominators as the numerator & the product as the denominator in the sum!

Since addition is commutative, swapping Fractions #1 & #2 will of course give you the same thing!

Fraction #1Fraction #2Sum
1/31/25/6
1/41/37/12
1/51/49/20
1/61/511/30
1/71/613/42
1/81/715/56
1/91/817/72
1/101/919/90
1/(x+1)1/x(2x+1)/(x^2+x)

Have you noticed that the numerators of the sums are consecutive odd numbers? That’s because odd numbers are the sums of 2 consecutive integers!

Back to Index Page Back to Math Trick Menu

© Derek Cumberbatch